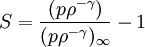Williams airfoil
From CFD-Wiki
| Line 1: | Line 1: | ||
| - | This test case consists of inviscid flow past the two-element Williams airfoil | + | This test case consists of inviscid flow past the two-element Williams airfoil for which exact potential solution is available. |
| - | ==Pressure coefficient on airfoil and flap== | + | In practice, the flow can be computed with a compressible solver at a low Mach number like 0.15 and zero angle of attack. Since the flow is inviscid, the drag coefficient must be close to zero and the entropy in the flow field must be constant. The lift coefficient is 3.736. Entropy production can be measured by computing |
| + | |||
| + | :<math> | ||
| + | S = \frac{(p \rho^{-\gamma})}{(p \rho^{-\gamma})_\infty} - 1 | ||
| + | </math> | ||
| + | |||
| + | ==Sample result== | ||
| + | The following results are obtained from a gridfree method called ''Kinetic Meshless Method''. The lift and drag coefficient are found to be 3.7608 and 0.0069 which is in good agreement with the exact values. The entropy variation in the computational domian is between <math>S_{\min}=-4.199 \times 10^{-4}</math> and <math>S_{\max}=7.246 \times 10^{-3}</math> | ||
| + | |||
| + | ===Pressure coefficient on airfoil and flap=== | ||
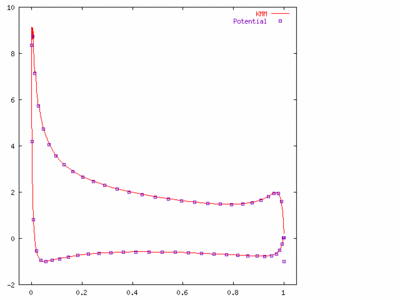
The pressure coefficient on the main airfoil and flap are compared with the exact potential solution. | The pressure coefficient on the main airfoil and flap are compared with the exact potential solution. | ||
Latest revision as of 06:29, 28 September 2005
This test case consists of inviscid flow past the two-element Williams airfoil for which exact potential solution is available.
In practice, the flow can be computed with a compressible solver at a low Mach number like 0.15 and zero angle of attack. Since the flow is inviscid, the drag coefficient must be close to zero and the entropy in the flow field must be constant. The lift coefficient is 3.736. Entropy production can be measured by computing
Sample result
The following results are obtained from a gridfree method called Kinetic Meshless Method. The lift and drag coefficient are found to be 3.7608 and 0.0069 which is in good agreement with the exact values. The entropy variation in the computational domian is between 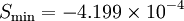 and
and 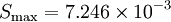
Pressure coefficient on airfoil and flap
The pressure coefficient on the main airfoil and flap are compared with the exact potential solution.