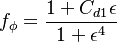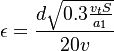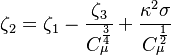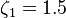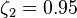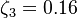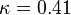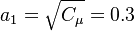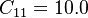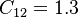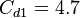Shuai-Agarwal turbulence Model
From CFD-Wiki
Lin.zhenghao (Talk | contribs) (Created page with "== Introduction == One-equation k-kL turbulence model was developed from two-equation k-kL closure of Abdol-Hamid et al. [1]. Improvements to the original formulation of one-equa...") |
m (→Shuai – Agarwal One Equation k-kL Turbulence Model) |
||
| (One intermediate revision not shown) | |||
| Line 1: | Line 1: | ||
== Introduction == | == Introduction == | ||
| - | One-equation k-kL turbulence model was developed from two-equation k-kL closure of Abdol-Hamid et al | + | One-equation k-kL turbulence model was developed from two-equation k-kL closure of Abdol-Hamid et al. Improvements to the original formulation of one-equation k-kL model (AIAA 2019-1879) were made by optimizing the model constants (AIAA 2020-1075). The new improved model has been validated by simulating several benchmark canonical wall-bounded turbulent flows with small regions of separation from NASA TMR (https://turbmodels.larc.nasa.gov/). The model needs further investigations by the scientific community to evaluate its potential. |
==Shuai – Agarwal One Equation k-kL Turbulence Model == | ==Shuai – Agarwal One Equation k-kL Turbulence Model == | ||
| - | '''The turbulent viscosity is given by:''' | + | '''The turbulent kinematic viscosity is given by:''' |
:<math> | :<math> | ||
| Line 19: | Line 19: | ||
:<math> | :<math> | ||
| - | P=\frac{\tau_{ij}}{\rho} \frac{\partial u_{i}}{\partial | + | P=\frac{\tau_{ij}}{\rho} \frac{\partial u_{i}}{\partial x_{j}} |
</math> | </math> | ||
| Line 29: | Line 29: | ||
:<math> | :<math> | ||
| - | S_{i j}=\frac{1}{2}\left(\frac{\partial u_{i}}{\partial | + | S_{i j}=\frac{1}{2}\left(\frac{\partial u_{i}}{\partial x_{j}} + \frac{\partial u_{j}}{\partial x_{i}} \right) |
</math> | </math> | ||
| Line 39: | Line 39: | ||
</math> | </math> | ||
| - | '''The | + | '''The parameters of the model are:''' |
:<math> | :<math> | ||
Latest revision as of 19:43, 10 April 2021
Introduction
One-equation k-kL turbulence model was developed from two-equation k-kL closure of Abdol-Hamid et al. Improvements to the original formulation of one-equation k-kL model (AIAA 2019-1879) were made by optimizing the model constants (AIAA 2020-1075). The new improved model has been validated by simulating several benchmark canonical wall-bounded turbulent flows with small regions of separation from NASA TMR (https://turbmodels.larc.nasa.gov/). The model needs further investigations by the scientific community to evaluate its potential.
Shuai – Agarwal One Equation k-kL Turbulence Model
The turbulent kinematic viscosity is given by:
The final form of the new Shuai-Agarwal one-equation k-kL model is obtained as:
Where 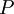 is the production term:
is the production term:
The following Bradshaw relation is used to express the relationship between  and k and kL:
and k and kL:
The parameters of the model are:
The model constants are:
References
- S. Shuai and R. K. Agarwal (2020), "A New Improved One-Equation Turbulence Model Based on k-kL Closure", AIAA Paper 2020-1075, January 2020.
- K. S. Abdol-Hamid, J. R. Carlson, and C. L. Rumsey (2016), "Verification and Validation of the k-kL Turbulence Model in FUN3D and CFL3D Codes", AIAA 2016-3941, 2016.

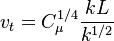
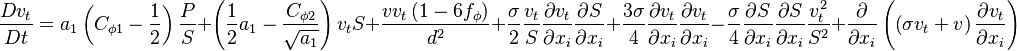

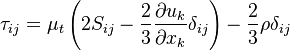
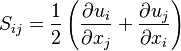
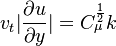

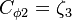
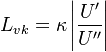
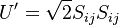
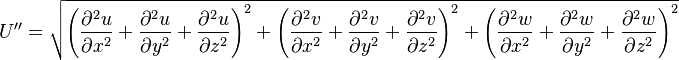
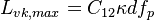
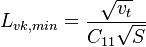
![f_{p}={min}{[max(\frac{P}{{\rho}{v_{t}}{S^{2}}} , 0.5) {,}{1.0}]}](/W/images/math/2/2/a/22a9d6e12e439928b712ce28fc78a45b.png)