CFD Online Logo CFD Online URL
www.cfd-online.com
[Sponsors]
Home > Wiki > Algebraic turbulence models

Algebraic turbulence models

From CFD-Wiki

(Difference between revisions)
Jump to: navigation, search
m (softened sentence)
 
(4 intermediate revisions not shown)
Line 1: Line 1:
-
Zero equation [[Turbulence modeling|turbulence models]] are models that do not require the solution of any additional equations, and are calculated directly from the flow variables. As a consequence, zero equation models may not be able to properly account for history effects on the turbulence, such as convection and diffusion of turbulent energy. These models are often too simple for use in general situations, but can be quite useful for simpler flow geometries or in start-up situations (e.g. the initial phases of a computation in which a more complicated model may have difficulties).  The two most well known zero equation models are the
+
{{Turbulence modeling}}
 +
 
 +
Algebraic [[Turbulence modeling|turbulence models]] or zero-equation turbulence models are models that do not require the solution of any additional equations, and are calculated directly from the flow variables. As a consequence, zero equation models may not be able to properly account for history effects on the turbulence, such as convection and diffusion of turbulent energy. These models are often too simple for use in general situations, but can be quite useful for simpler flow geometries or in start-up situations (e.g. the initial phases of a computation in which a more complicated model may have difficulties).  The two most well known zero equation models are the
*[[Baldwin-Lomax model]] and the
*[[Baldwin-Lomax model]] and the
Line 5: Line 7:
Other even simpler models, such a models written as <math>\mu_t = f(y^+)</math>, are sometimes used in particular situations (e.g. boundary layers or jets).
Other even simpler models, such a models written as <math>\mu_t = f(y^+)</math>, are sometimes used in particular situations (e.g. boundary layers or jets).
 +
 +
The algebraic [[Johnson-King model]] is sometimes called a 1/2 equation model since it incorporates the solution of an ordinary differential equation.
 +
 +
[[Category: Turbulence models]]

Latest revision as of 20:39, 1 April 2007

Turbulence modeling
Turbulence
RANS-based turbulence models
  1. Linear eddy viscosity models
    1. Algebraic models
      1. Cebeci-Smith model
      2. Baldwin-Lomax model
      3. Johnson-King model
      4. A roughness-dependent model
    2. One equation models
      1. Prandtl's one-equation model
      2. Baldwin-Barth model
      3. Spalart-Allmaras model
    3. Two equation models
      1. k-epsilon models
        1. Standard k-epsilon model
        2. Realisable k-epsilon model
        3. RNG k-epsilon model
        4. Near-wall treatment
      2. k-omega models
        1. Wilcox's k-omega model
        2. Wilcox's modified k-omega model
        3. SST k-omega model
        4. Near-wall treatment
      3. Realisability issues
        1. Kato-Launder modification
        2. Durbin's realizability constraint
        3. Yap correction
        4. Realisability and Schwarz' inequality
  2. Nonlinear eddy viscosity models
    1. Explicit nonlinear constitutive relation
      1. Cubic k-epsilon
      2. EARSM
    2. v2-f models
      1. \overline{\upsilon^2}-f model
      2. \zeta-f model
  3. Reynolds stress model (RSM)
Large eddy simulation (LES)
  1. Smagorinsky-Lilly model
  2. Dynamic subgrid-scale model
  3. RNG-LES model
  4. Wall-adapting local eddy-viscosity (WALE) model
  5. Kinetic energy subgrid-scale model
  6. Near-wall treatment for LES models
Detached eddy simulation (DES)
Direct numerical simulation (DNS)
Turbulence near-wall modeling
Turbulence free-stream boundary conditions
  1. Turbulence intensity
  2. Turbulence length scale

Algebraic turbulence models or zero-equation turbulence models are models that do not require the solution of any additional equations, and are calculated directly from the flow variables. As a consequence, zero equation models may not be able to properly account for history effects on the turbulence, such as convection and diffusion of turbulent energy. These models are often too simple for use in general situations, but can be quite useful for simpler flow geometries or in start-up situations (e.g. the initial phases of a computation in which a more complicated model may have difficulties). The two most well known zero equation models are the

Other even simpler models, such a models written as 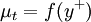\mu_t = f(y^+), are sometimes used in particular situations (e.g. boundary layers or jets).

The algebraic Johnson-King model is sometimes called a 1/2 equation model since it incorporates the solution of an ordinary differential equation.

Retrieved from "https://mail.cfd-online.com/Wiki/Algebraic_turbulence_models"
My wiki