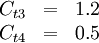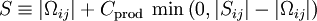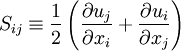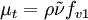Spalart-Allmaras model
From CFD-Wiki
Littleandy (Talk | contribs) m (Category added) |
(Corrected an error) |
||
| (8 intermediate revisions not shown) | |||
| Line 1: | Line 1: | ||
| - | + | {{Turbulence modeling}} | |
| + | Spalart-Allmaras model is a one equation model which solves a transport equation for a viscosity-like variable <math>\tilde{\nu}</math>. This may be referred to as the Spalart-Allmaras variable. | ||
== Original model == | == Original model == | ||
| Line 9: | Line 10: | ||
:<math> | :<math> | ||
| - | \frac{\partial \tilde{\nu}}{\partial t} + u_j \frac{\partial \tilde{\nu}}{\partial x_j} = C_{b1} [1 - f_{t2}] \tilde{S} \tilde{\nu} + \frac{1}{\sigma} \{ \nabla \cdot [(\nu + \tilde{\nu}) \nabla \tilde{\nu}] + C_{b2} | \nabla \nu |^2 \} - \left[C_{w1} f_w - \frac{C_{b1}}{\kappa^2} f_{t2}\right] \left( \frac{\tilde{\nu}}{d} \right)^2 + f_{t1} \Delta U^2 | + | \begin{matrix} |
| + | \frac{\partial \tilde{\nu}}{\partial t} + u_j \frac{\partial \tilde{\nu}}{\partial x_j} & = & C_{b1} [1 - f_{t2}] \tilde{S} \tilde{\nu} + \frac{1}{\sigma} \{ \nabla \cdot [(\nu + \tilde{\nu}) \nabla \tilde{\nu}] + C_{b2} | \nabla \tilde{\nu} |^2 \} - \\ | ||
| + | \ & \ & \left[C_{w1} f_w - \frac{C_{b1}}{\kappa^2} f_{t2}\right] \left( \frac{\tilde{\nu}}{d} \right)^2 + f_{t1} \Delta U^2 \\ | ||
| + | \end{matrix} | ||
</math> | </math> | ||
| Line 15: | Line 19: | ||
\tilde{S} \equiv S + \frac{ \tilde{\nu} }{ \kappa^2 d^2 } f_{v2}, \quad f_{v2} = 1 - \frac{\chi}{1 + \chi f_{v1}} | \tilde{S} \equiv S + \frac{ \tilde{\nu} }{ \kappa^2 d^2 } f_{v2}, \quad f_{v2} = 1 - \frac{\chi}{1 + \chi f_{v1}} | ||
</math> | </math> | ||
| + | |||
| + | where | ||
| + | |||
| + | :<math> | ||
| + | S = \equiv \sqrt{2 \Omega_{ij} \Omega_{ij}} | ||
| + | </math> | ||
| + | |||
| + | :<math>\Omega_{ij} \equiv \frac{1}{2} ( \frac{\partial u_i}{\partial x_j} - \frac{\partial u_j}{\partial x_i} )</math> | ||
:<math> | :<math> | ||
| Line 27: | Line 39: | ||
f_{t2} = C_{t3} \exp(-C_{t4} \chi^2) | f_{t2} = C_{t3} \exp(-C_{t4} \chi^2) | ||
</math> | </math> | ||
| + | |||
| + | :d is the distance to the closest surface | ||
The constants are | The constants are | ||
| Line 55: | Line 69: | ||
\end{matrix} | \end{matrix} | ||
</math> | </math> | ||
| + | |||
| + | [Dacles-Mariani et. al. 1995] proposed a modification of the model which also accounts for the effect of mean strain rate on turbulence production. This modification instead prescribes: | ||
| + | |||
| + | :<math> | ||
| + | S \equiv |\Omega_{ij}| + C_{\rm prod} \; \min \left(0, |S_{ij}| - |\Omega_{ij}| \right) | ||
| + | </math> | ||
| + | |||
| + | where | ||
| + | |||
| + | :<math>C_{\rm prod} = 2.0</math> | ||
| + | :<math>|\Omega_{ij}| \equiv \sqrt{2 \Omega_{ij} \Omega_{ij}}</math> | ||
| + | :<math>|S_{ij}| \equiv \sqrt{2 S_{ij} S_{ij}}</math> | ||
| + | :<math>\Omega_{ij} \equiv \frac{1}{2}\left(\frac{\partial u_j}{\partial x_i} - \frac{\partial u_i}{\partial x_j} \right)</math> | ||
| + | :<math>S_{ij} \equiv \frac{1}{2}\left(\frac{\partial u_j}{\partial x_i} + \frac{\partial u_i}{\partial x_j} \right)</math> | ||
Other models related to the S-A model: | Other models related to the S-A model: | ||
| Line 78: | Line 106: | ||
== Boundary conditions == | == Boundary conditions == | ||
| - | + | Boundary conditions are set by defining values of <math>\tilde{\nu}</math>. | |
| - | [[ | + | Freestream boundary conditions are discussed in [[Turbulence free-stream boundary conditions|turbulence free-stream boundary conditions]]. |
| - | + | Walls: <math>\tilde{\nu}=0</math> | |
| + | |||
| + | Outlet: convective outlet. | ||
== References == | == References == | ||
| + | |||
| + | * {{reference-paper|author=Dacles-Mariani, J., Zilliac, G. G., Chow, J. S. and Bradshaw, P.|year=1995|title=Numerical/Experimental Study of a Wingtip Vortex in the Near Field|rest=AIAA Journal, 33(9), pp. 1561-1568, 1995}} | ||
* {{reference-paper|author=Spalart, P. R. and Allmaras, S. R.|year=1992|title=A One-Equation Turbulence Model for Aerodynamic Flows|rest=AIAA Paper 92-0439}} | * {{reference-paper|author=Spalart, P. R. and Allmaras, S. R.|year=1992|title=A One-Equation Turbulence Model for Aerodynamic Flows|rest=AIAA Paper 92-0439}} | ||
Latest revision as of 13:34, 23 April 2015
Spalart-Allmaras model is a one equation model which solves a transport equation for a viscosity-like variable 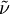 . This may be referred to as the Spalart-Allmaras variable.
. This may be referred to as the Spalart-Allmaras variable.
Contents |
Original model
The turbulent eddy viscosity is given by
where
- d is the distance to the closest surface
The constants are
Modifications to original model
According to Spalart it is safer to use the following values for the last two constants:
[Dacles-Mariani et. al. 1995] proposed a modification of the model which also accounts for the effect of mean strain rate on turbulence production. This modification instead prescribes:
where
Other models related to the S-A model:
DES (1999) [1]
DDES (2006)
Model for compressible flows
There are two approaches to adapting the model for compressible flows. In the first approach the turbulent dynamic viscosity is computed from
where 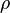 is the local density. The convective terms in the equation for
is the local density. The convective terms in the equation for 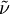 are modified to
are modified to
where the right hand side (RHS) is the same as in the original model.
Boundary conditions
Boundary conditions are set by defining values of 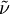 .
.
Freestream boundary conditions are discussed in turbulence free-stream boundary conditions.
Walls: 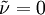
Outlet: convective outlet.
References
- Dacles-Mariani, J., Zilliac, G. G., Chow, J. S. and Bradshaw, P. (1995), "Numerical/Experimental Study of a Wingtip Vortex in the Near Field", AIAA Journal, 33(9), pp. 1561-1568, 1995.
- Spalart, P. R. and Allmaras, S. R. (1992), "A One-Equation Turbulence Model for Aerodynamic Flows", AIAA Paper 92-0439.
- Spalart, P. R. and Allmaras, S. R. (1994), "A One-Equation Turbulence Model for Aerodynamic Flows", La Recherche Aerospatiale n 1, 5-21.

 model
model
 model
model

![\begin{matrix}
\frac{\partial \tilde{\nu}}{\partial t} + u_j \frac{\partial \tilde{\nu}}{\partial x_j} & = & C_{b1} [1 - f_{t2}] \tilde{S} \tilde{\nu} + \frac{1}{\sigma} \{ \nabla \cdot [(\nu + \tilde{\nu}) \nabla \tilde{\nu}] + C_{b2} | \nabla \tilde{\nu} |^2 \} - \\
\ & \ & \left[C_{w1} f_w - \frac{C_{b1}}{\kappa^2} f_{t2}\right] \left( \frac{\tilde{\nu}}{d} \right)^2 + f_{t1} \Delta U^2 \\
\end{matrix}](/W/images/math/f/d/e/fdec625b1779e143145244581d4b32ec.png)

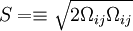

![f_w = g \left[ \frac{ 1 + C_{w3}^6 }{ g^6 + C_{w3}^6 } \right]^{1/6}, \quad g = r + C_{w2}(r^6 - r), \quad r \equiv \frac{\tilde{\nu} }{ \tilde{S} \kappa^2 d^2 }](/W/images/math/0/9/b/09b19885ed6dffe3dec850e2516f1696.png)
![f_{t1} = C_{t1} g_t \exp\left( -C_{t2} \frac{\omega_t^2}{\Delta U^2} [ d^2 + g^2_t d^2_t] \right)](/W/images/math/9/9/3/993b41d0ba795e0b3877d041c4cff1cb.png)