Mach number
From CFD-Wiki
(Difference between revisions)
m |
|||
| Line 4: | Line 4: | ||
:<math>M \equiv \frac{u}{a} \equiv \frac{\mbox{local flow speed}}{\mbox{local speed of sound}}</math> | :<math>M \equiv \frac{u}{a} \equiv \frac{\mbox{local flow speed}}{\mbox{local speed of sound}}</math> | ||
| - | The Mach number can be used to categorise compressible flows into different [[Mach number regimes]] | + | The Mach number can be used to categorise compressible flows into different [[Mach number regimes]]. To get a more physical insight into Mach number, it is useful if it is viewed as being proportional to the ratio of Kinetic energy to the internal energy of the molecules. |
The name "Mach number" originates from the famous pioneer in compressible fluid dynamics [[Ernst Mach]] | The name "Mach number" originates from the famous pioneer in compressible fluid dynamics [[Ernst Mach]] | ||
[[Category: Dimensionless parameters]] | [[Category: Dimensionless parameters]] | ||
Revision as of 19:07, 30 September 2005
The Mach number,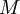 
, is the local flow-speed, 
, divided by the local
speed of sound,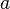 
:
The Mach number can be used to categorise compressible flows into different Mach number regimes. To get a more physical insight into Mach number, it is useful if it is viewed as being proportional to the ratio of Kinetic energy to the internal energy of the molecules.
The name "Mach number" originates from the famous pioneer in compressible fluid dynamics Ernst Mach
