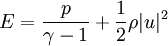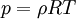Inviscid flow
From CFD-Wiki
(→Related pages) |
(→Euler equations as the limit of Navier-Stokes equations) |
||
| Line 50: | Line 50: | ||
Have a look at this diskussion: | Have a look at this diskussion: | ||
http://www.cfd-online.com/Forum/fluent.cgi?read=32738 | http://www.cfd-online.com/Forum/fluent.cgi?read=32738 | ||
| + | http://www.cfd-online.com/Forum/fluent.cgi?read=32788 | ||
==Related pages== | ==Related pages== | ||
Revision as of 12:00, 15 November 2005
A flow in which viscous effects can be neglected is known as inviscid flow. At high Reynolds numbers, flow past slender bodies involve thin boundary layers. Viscous effects are important only inside the boundary layer and the flow outside it is nearly inviscid. If the boundary layer is not separated then the inviscid flow model can be used to predict the pressure distribution with reasonable accuracy. Although no practical flow is inviscid, the inviscid assumption is valid if the time scales for diffusion are much larger compared to the time scales for convection, which is measured by the Reynolds number.
Governing Equations
The governing equations for inviscid flow, also known as Euler equations, are obtained by discarding the viscous terms from the Navier-Stokes equations
- Continuity equation
- Momentum equation
- Energy equation
where
-
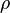 is the density
is the density
-
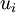 is the fluid velocity
is the fluid velocity
-
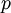 is the pressure
is the pressure
-
 is the total energy per unit mass of fluid
is the total energy per unit mass of fluid
-
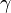 is the ratio of specific heats
is the ratio of specific heats
The above equations are closed by taking an equation of state, the simplest being the ideal gas
where
-
 is the gas constant
is the gas constant
-
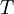 is the absolute temperature
is the absolute temperature
Have a look at this diskussion: http://www.cfd-online.com/Forum/fluent.cgi?read=32738 http://www.cfd-online.com/Forum/fluent.cgi?read=32788


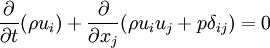
![\frac{\partial}{\partial t}(\rho E) + \frac{\partial}{\partial x_j}[(\rho E + p)u_j] = 0](/W/images/math/e/7/3/e73b09e7ac9cb3a2f73034d30bfbcb11.png)