K-omega models
From CFD-Wiki
(Difference between revisions)
Tohoughton (Talk | contribs) (→Introduction) |
|||
| Line 3: | Line 3: | ||
The K-omega model is one of the most common [[Turbulence modeling|turbulence models]]. It is a [[Two equation models|two equation model]], that means, it includes two extra transport equations to represent the turbulent properties of the flow. This allows a two equation model to account for history effects like convection and diffusion of turbulent energy. | The K-omega model is one of the most common [[Turbulence modeling|turbulence models]]. It is a [[Two equation models|two equation model]], that means, it includes two extra transport equations to represent the turbulent properties of the flow. This allows a two equation model to account for history effects like convection and diffusion of turbulent energy. | ||
| + | |||
The first transported variable is turbulent kinetic energy, <math>k</math>. The second transported variable in this case is the specific dissipation, <math>\omega</math>. It is the variable that determines the scale of the turbulence, whereas the first variable, <math>k</math>, determines the energy in the turbulence. | The first transported variable is turbulent kinetic energy, <math>k</math>. The second transported variable in this case is the specific dissipation, <math>\omega</math>. It is the variable that determines the scale of the turbulence, whereas the first variable, <math>k</math>, determines the energy in the turbulence. | ||
| + | |||
| + | To calculate boundary conditions for this model see [[Turbulence free-stream boundary conditions|turbulence free-stream boundary conditions]]. | ||
== Common used K-omega models == | == Common used K-omega models == | ||
Revision as of 09:16, 15 May 2008
Introduction
The K-omega model is one of the most common turbulence models. It is a two equation model, that means, it includes two extra transport equations to represent the turbulent properties of the flow. This allows a two equation model to account for history effects like convection and diffusion of turbulent energy.
The first transported variable is turbulent kinetic energy, 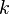 . The second transported variable in this case is the specific dissipation,
. The second transported variable in this case is the specific dissipation, 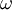 . It is the variable that determines the scale of the turbulence, whereas the first variable,
. It is the variable that determines the scale of the turbulence, whereas the first variable, 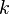 , determines the energy in the turbulence.
, determines the energy in the turbulence.
To calculate boundary conditions for this model see turbulence free-stream boundary conditions.

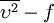 model
model
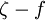 model
model