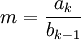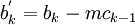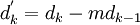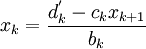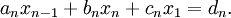Tridiagonal matrix algorithm - TDMA (Thomas algorithm)
From CFD-Wiki
Contents |
Introduction
The tridiagonal matrix algorithm (TDMA), also known as the Thomas algorithm, is a simplified form of Gaussian elimination that can be used to solve tridiagonal systems of equations. A tridiagonal system may be written as
where 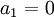 and
and 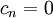 . In matrix form, this system is written as
. In matrix form, this system is written as
For such systems, the solution can be obtained in 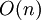 operations instead of
operations instead of  required by Gaussian Elimination. A first sweep eliminates the
required by Gaussian Elimination. A first sweep eliminates the  's, and then an (abbreviated) backward substitution produces the solution. Example of such matrices commonly arise from the discretization of 1D problems (e.g. the 1D Possion problem).
's, and then an (abbreviated) backward substitution produces the solution. Example of such matrices commonly arise from the discretization of 1D problems (e.g. the 1D Possion problem).
Algorithm
Forward elimination phase
- for k = 2 step until n do
-
- end loop (k)
Backward substitution phase
-
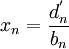
- for k = n-1 stepdown until 1 do
-
- end loop (k)
Variants
In some situations, particularly those involving periodic boundary conditions, a slightly perturbed form of the tridiagonal system may need to be solved:
In this case, we can make use of the Sherman-Morrison formula to avoid the additional operations of Gaussian elimination and still use the Thomas algorithm.
In other situation, the system of equation may be block tridiagonal, with smaller submatrices arranged as the individual elements in the above matrix system. Simplified forms of Gaussian elimination have been developed for these situations.
References
- Conte, S.D., and deBoor, C. (1972), Elementary Numerical Analysis, McGraw-Hill, New York..
TODO: Add more references, more on the variants, and maybe more performance type info
--Jasond 16:50, 18 December 2005 (MST)

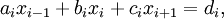
![\left[
\begin{matrix}
{b_1} & {c_1} & { } & { } & { 0 } \\
{a_2} & {b_2} & {c_2} & { } & { } \\
{ } & {a_3} & {b_3} & \cdot & { } \\
{ } & { } & \cdot & \cdot & {c_{n-1}}\\
{ 0 } & { } & { } & {a_n} & {b_n}\\
\end{matrix}
\right]
\left[
\begin{matrix}
{x_1 } \\
{x_2 } \\
\cdot \\
\cdot \\
{x_n } \\
\end{matrix}
\right]
=
\left[
\begin{matrix}
{b_1 } \\
{b_2 } \\
\cdot \\
\cdot \\
{b_n } \\
\end{matrix}
\right].](/W/images/math/9/1/0/910153779e83377fcdfb2309efcda195.png)