Introduction to turbulence/Study questions

From CFD-Wiki

Questions related to the nature of turbulence

1. Observe your surroundings carefully and identify at least ten different turbulent phenomena for which you can actually see flow patterns. Write down what you find particularly interesting about each.
2. Talk to people (especially engineers) you know (or even don’t know particularly well) about what they think the turbulence problem is. Decide for yourself whether they have fallen into the trap that Professor Jones talks about in the quotation used in this text.
3. Some believe that computers have already (or at least soon will) make experiments in turbulence unnecessary. The simplest flow one can imagine of sufficiently high Reynolds number to really test any of the theoretical ideas about turbulence will require a computational box of approximately 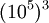, because of the large range of scales needed. The largest simulation to-date uses a computational box of 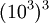, and takes several thousand hours of processor time. Assuming computer capacity continues to double every 1.5 years, calculate how many years it will be before even this simple experiment can be done in a computer.
4. The famous aerodynamicist Theodore von Karman once said: “A scientist studies what is; an engineer creates what has never been.” Think about this in the context of the comments in Chapter 1, and about the differing goals of the scientist and the engineer. Then try to figure out how you can plot a life course that will not trap you into thinking your own little corner of the world is all there is.
5. Think about the comments that ideas become accepted simply because they have been around awhile without being disproved. Can you think of examples from history, or from your own personal experience? Why do you think this happens? And how can we avoid it, at least in our work as scientists and engineers?

Questions related to statistical analysis

1. By using the definition of the probability density function as the limit of the histogram of a random variable as the internal size goes to zero and as the number of realizations becomes infinite, show that the probability average defined by 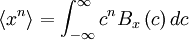and the ensemble average defined by are the same.
2. By completing the square in the exponential, prove that the pdf for the normal distribution given by 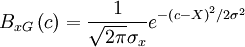integrates to unity.
3. Prove the equation
4. Prove for a normal distribution that the skewness is equal to zero and that the kurtosis is equal to three.
5. Show by integrating over one of the variables that the Gaussian jpdf given by integrates to the marginal distribution pdf given by 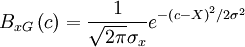, regardless of the value of the correlation coefficient.
6. Find the variability of an estimator for the variance using the equation , but with the sample mean, XN, substituted for the true mean, X.
7. Create a simple estimator for the fourth central moment, assuming the second to be known exactly. Then find its variability for a Gaussian distributed random variable.
8. You are attempting to measure a Gaussian distributed random variable with 12 bit A/D converter which can only accept voltage inputs between 0 and
9. Assume the mean voltage is +4, and the rms voltage is 4. Show what a histogram of your measured signal would look like assuming any voltage which is clipped goes into the first or last bins. Also compute the first three moments (central) of the measured signal.

Credits

This text was based on "Lectures in Turbulence for the 21st Century" by Professor William K. George, Professor of Turbulence, Chalmers University of Technology, Gothenburg, Sweden.